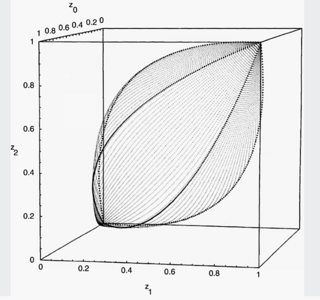
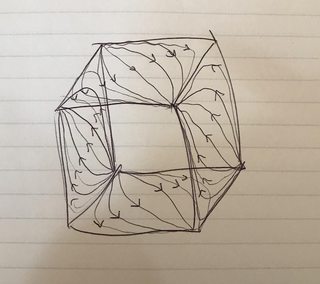
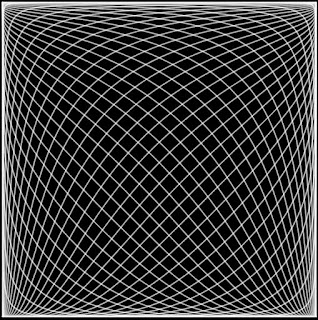
Gluing leaves in zeta space and automorphisms on the resulting quotient spaces
Define an analytic planar homotopy $h_t: (0,1) \to (0,1)$ for $t\in[1/3,3]$ where $h_t(x)=e^{\frac{t}{\log x}}$ s.t. $h_{1/3}=e^{\frac{1/3}{\log x}}$ and $h_3=e^{\frac{3}{\log x}}$ for $x\in(0,1)$. I want a rotational homotopy that projects onto the planar homotopy. So, define the equivalence relation on the boundary curves of the closed subset of $(0,1)^2$, that is $h_{1/3}\sim h_3$, where points are identified by straight lines with slope $1$, connecting $h_{1/3}$ and $h_3$. This yields a spherical topology $\mathcal S$ embedded in $(0,1)^3$ with the endpoints of the sphere being singularities at $p=(0,1,1)$ and $q=(1,0,0)$. Conceptually it's clear that a projection $\pi: (0,1)^3 \to (0,1)^2$ takes the leaves on $\mathcal S$ to $h_t(x)$ as a double cover. But my main objective of study is to put a metric on $\mathcal S$. The $1$-parameter metric I derived on the planar space is $g_t(r)=\int_{(0,1)} x^{r-1}h_t(x)~dx = 2\sqrt{\frac{t}{r}}K_1(2\sqrt{tr})$ and it measures the distan