A description of $\zeta-$space

I would like to attempt to define a mathematical space I call $\zeta-$space.  $\zeta-$space has a description as the transversal intersection of two isometric pseudo-Riemannian manifolds (Lorentzian submanifolds) both equipped with the Lorentz metric. It is somewhat laborious to describe and derive $\zeta$ because it is not a standard approach and $\zeta$ does not have a clean representation so to speak. However it is a fundamentally important mathematical and physical object in its own right and should be studied.

 I define $\zeta$ in the following way:

 $\zeta:=\{\varphi_S \} \cap \{ \varphi_T \}$

 Under this first level description, $\zeta$ is the intersection between two class structures denoted as $\varphi_S$ and $\varphi_T.$ These class structures can be thought of as a family of functions and also as lines of constant time and constant space respectively:

 $$ \varphi_S(x) = e^{\frac{S}{\log(x)}} $$

 $$ \varphi_T(x) = e^{\frac{T}{\log(1-x)}}$$

 Here I view $S,T$ as fundamental generators. That is, they completely generate the space. Here is how I derived them and began to think of them as generators:

 I set $\varphi_S(x)=x$ and solved for $S.$ I got $S=\{\log^2(x) : x \in \Bbb R \cap (0,1) \}.$ However I thought it better to replace $x$ with some parameter $s.$ So, $S=\{\log^2(s) : s \in \Bbb R \cap (0,1) \}.$ Likewise I set $\varphi_T(x)=1-x$ and solved for $T.$

 Here we have a visualization of $\zeta$ showing the overlapping class structures $\{ \varphi_S\}$ and $\{\varphi_T\}:$

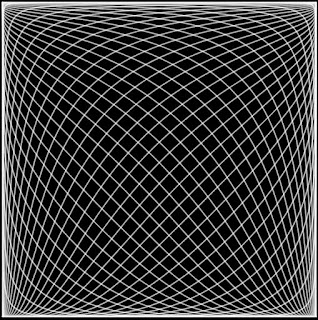$\zeta-$space as the intersection of two Lorentzian submanifolds


Comments

Post a Comment

Popular posts from this blog

Automorphisms and zeta space

Image
Here I will operate under the assumption that $\phi_s$ is a unique and well-posed solution to the following PDE. This means whatever conditions are needed for that to occur, will be imposed. $$s\frac{\partial^2}{\partial s^2}\phi_s(x)=\mp x\frac{\partial}{\partial x}\phi_s(x) $$ for which we have our pre-assigned unique solution: $$\mathcal \phi_{s}:=\bigg\lbrace e^{\frac{\pm s}{\log x}}:s \in \Bbb R \bigg\rbrace$$ We have singularities at $s=0$ and $x=0,1$ and this will be called a "cross singularity" or  $CS$. A natural idea would be to intialize some distribution on this $CS$ and run time forward giving a wellposed analytic solution for all time. So we choose our pre-assigned solution $\phi_s$ and run the solution back in time where we obtain what we wanted, at least qualitatively. We can throw out our solution for $s<0$ because it doesn't directly apply to the situation, and it does not rapidly decay. Meanwhile, for $s>0$ it is Schwartz i.e. has a rapid decay cr...
Read more

Gluing leaves in zeta space and automorphisms on the resulting quotient spaces

Define an analytic planar homotopy $h_t: (0,1) \to (0,1)$ for $t\in[1/3,3]$ where $h_t(x)=e^{\frac{t}{\log x}}$ s.t. $h_{1/3}=e^{\frac{1/3}{\log x}}$ and $h_3=e^{\frac{3}{\log x}}$ for $x\in(0,1)$. I want a rotational homotopy that projects onto the planar homotopy. So, define the equivalence relation on the boundary curves of the closed subset of $(0,1)^2$, that is $h_{1/3}\sim h_3$, where points are identified by straight lines with slope $1$, connecting $h_{1/3}$ and $h_3$. This yields a spherical topology $\mathcal S$ embedded in $(0,1)^3$ with the endpoints of the sphere being singularities at $p=(0,1,1)$ and $q=(1,0,0)$.  Conceptually it's clear that a projection $\pi: (0,1)^3 \to (0,1)^2$ takes the leaves on $\mathcal S$ to $h_t(x)$ as a double cover. But my main objective of study is to put a metric on $\mathcal S$. The $1$-parameter metric I derived on the planar space is $g_t(r)=\int_{(0,1)} x^{r-1}h_t(x)~dx = 2\sqrt{\frac{t}{r}}K_1(2\sqrt{tr})$ and it measures the distan...
Read more